Two-tap equalizer with four-tap channel and BPSK source

Next: Two-tap equalizer with six-tap Up: Simulation Results Previous: Simulation Results

3.1 Two-tap equalizer with four-tap channel and BPSK source

Consider the communications system in Figure 1 with an i.i.d. BPSK source, the channel $\ensuremath{\mathbf{c}}=\left[\begin{array} {cccc}0.2&0.5&1&-0.1\end{array}\right]^T$ , and a two-tap FSE (N_f=2).

**Figure 2:** SE-CMA cost contours for BPSK source, 15 dB SNR
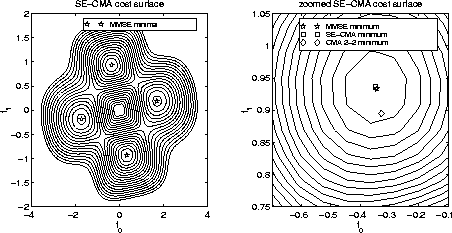$\begin{figure} \begin{center} \epsfxsize=4in \epsfbox{ex1.eps} \end{center} \end{figure}$

Figure 2a shows the SE-CMA cost contours for this system when the SNR is 15 dB. Figure 2b is a zoomed version of Figure 2a that shows the locations of the optimal MMSE, SE-CMA, and CMA 2-2 minima overlaid on the SE-CMA cost surface. As can be seen, the SE-CMA minimum is closer to the MMSE minimum than is CMA 2-2.

A numeric simulation was also performed to prove that the SE-CMA equalizer attains lower error than the CMA 2-2 equalizer. For 20,000 iterations with 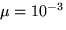$\mu=10^{-3}$ , a portion of the squared-error history is shown in Figure 3.

**Figure 3:** Comparison of error history for SE-CMA and CMA 2-2 (BPSK, SNR=15dB)
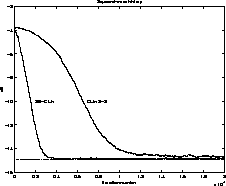$\begin{figure} \begin{center} \epsfxsize=2in \epsfbox{ex1b.eps} \end{center} \end{figure}$

The SE-CMA error levels off at about -14.85 dB, whereas the CMA 2-2 error only gets to about -14.65 dB. Therefore, I have shown for this BPSK system that the more efficient SE-CMA is superior to the more common CMA 2-2.

Next: Two-tap equalizer with six-tap Up: Simulation Results Previous: Simulation Results

Andrew Grant Klein
8/12/1998