Two-tap equalizer with four-tap channel and 8-PAM source

Next: More on the simulations. Up: Simulation Results Previous: 32-tap equalizer with 64-tap

3.4 Two-tap equalizer with four-tap channel and 8-PAM source

Now consider the same communications system, but with symbols chosen from a unit-variance 8-PAM alphabet, the channel given by $\ensuremath{\mathbf{c}}=\left[\begin{array} {cccc}0.2&0.5&1&-0.1\end{array}\right]^T$ , and a two-tap FSE (N_f=2).

**Figure 8:** SE-CMA cost contours with 8-PAM source, 15 dB SNR
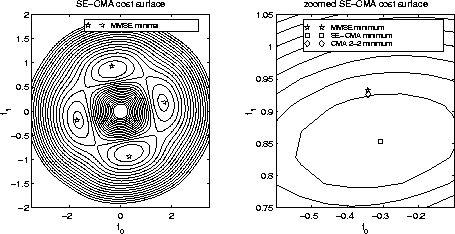$\begin{figure} \begin{center} \epsfxsize=4in \epsfbox{ex3.eps} \end{center} \end{figure}$

Figure 8 shows the SE-CMA cost contours for this system when the SNR is 15 dB. However, the SE-CMA minimum is now further from the MMSE minimum than is CMA 2-2.

For 70,000 iterations with 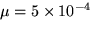$\mu=5\times10^{-4}$ , a portion of the squared-error history for a simulation is shown in Figure 9.

**Figure 9:** Comparison of error history for SE-CMA and CMA 2-2 with 8-PAM source
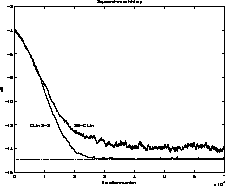$\begin{figure} \begin{center} \epsfxsize=2in \epsfbox{ex3b.eps} \end{center} \end{figure}$

As shown, CMA 2-2 now attains a lower error than SE-CMA. Therefore, it has been shown that SE-CMA is inferior for anything more complicated than BPSK sources. I tried various simulations with other more complicated alphabets such as 4-PAM, 16-PAM, etc., and found that CMA 2-2 performs better in these cases as well.

Next: More on the simulations. Up: Simulation Results Previous: 32-tap equalizer with 64-tap

Andrew Grant Klein
8/12/1998